

The Clifford group is generated by three gates, Hadamard, phase gate S, and CNOT.

Taking an average over a finite group G (like the Clifford group) of a.
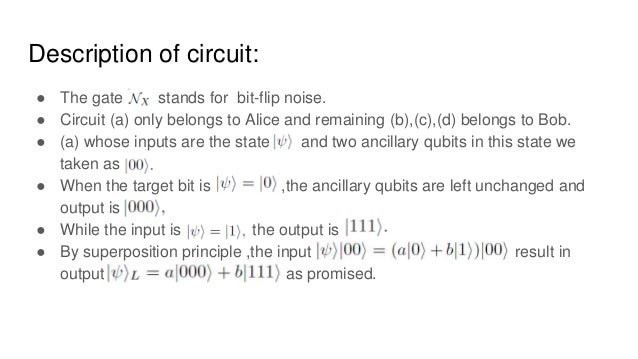
#Clifford group quantum error correction code#
Quantum circuits that consist of only Clifford gates can be efficiently simulated with a classical computer due to the Gottesman–Knill theorem. groups for the 3 bit bit-flip error correction code encoded subspace. Multiple-particle interference and quantum error correction. An introduction to quantum error correction and fault-tolerant quantum. Here we revisit this theorem and extend it to quantum circuits composed of Clifford and T gates, where T is the single-qubit 45-degree phase shift. The notion was introduced by Daniel Gottesman and is named after the mathematician William Kingdon Clifford. Clifford group is incapable of universal quantum computation (assuming BPP BQP). This suggests that the Clifford-twisted automorphism group might be the correct auto-. The Gottesman-Knill theorem asserts that a quantum circuit composed of Clifford gates can be efficiently simulated on a classical computer. In quantum computing and quantum information theory, the Clifford gates are the elements of the Clifford group, a set of mathematical transformations which normalize the n-qubit Pauli group, i.e., map tensor products of Pauli matrices to tensor products of Pauli matrices through conjugation.


 0 kommentar(er)
0 kommentar(er)
